For this lab experiment, you will attempt to determine the constant acceleration all objects in freefall feel due to the Earth's gravity.
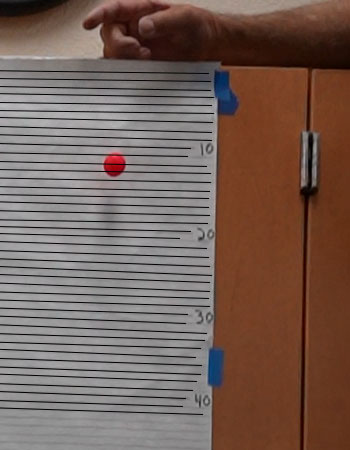
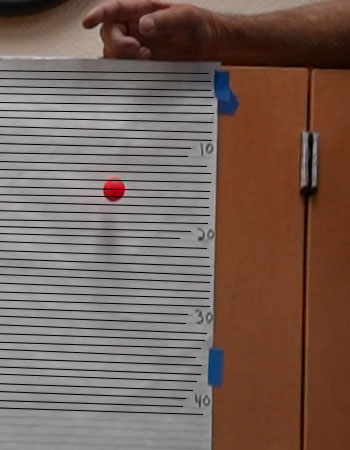
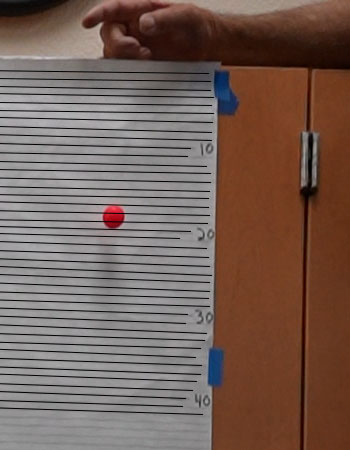
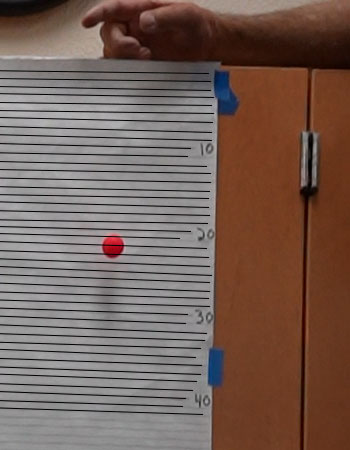
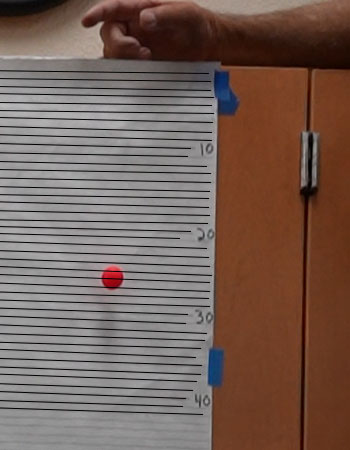
The following images are close-up views of a portion of some of the individual frames taken from the video of one of the balls being dropped as you saw in the demonstration video you watched for this lab exercise. Since the ball is continually in motion the entire time, even during the short period of time each video frame is being captured and in between the times when each new video frame is captured, its velocity you calculate will therefore be its average velocity it experienced during that period of time, not its instantaneous velocity at some specific instance in time. You will only be looking at the first few frames immediately following the release of the ball while its velocity is still low enough that it remains relatively near to the starting point so we can zoom in on a small region to allow you to more easily measure its position in each frame.
You can label the position and time when the ball is at the top as '0'. This occurs in the very first frame (Frame 0) you see. Because it's possible the ball was released during or just prior to this frame being captured, don't assume its velocity is zero in frame 0. To calculate the average velocity during the change between any two subsequent frames you will take the difference in position between those 2 frames and divide it by the difference in time between those 2 frames. To get the difference in position you will need to estimate the ball's position in each frame. To get the difference in time you simply need to know that the frame rate for these images is 60 frames per second, and so the inverse of this value tells you how many seconds pass in between subsequent frames.
Once you have the average velocity determined between each subsequent pair of frames for all the frames shown, you can now calculate the average accleration between any two subsequent frames in a similar manner to how you calculated average velocity, except you will use the difference in velocity between 2 subsequent frames instead of the difference in position. The time difference between the 2 frames is the same as it is for your velocity calculations
Recognizing that the acceleration should be constant (i.e. it never changes - it always has the same value), since it's the constant acceleration due to gravity on Earth g, it should stand to reason that your average acceleration should be equal to the constant instantaneous acceleration g. However, experiments always have many typical sources of error in them - from how the ball is falling, to how the photo is being captured, to how you are measuring its position - so it's very likely that many of your values will not be g, and may turn out significantly different than g. But, if you calculate an overall average using all your individual frame pair average acceleration values, that will give you many data points to help smooth out any errors and presumably the result should be in the ballpark of g, as expected.